Simulation von RT-CVD für Graphen und andere zwei-dimensionale Materialien
The epitaxial growth of graphene is influenced by the interaction of graphene with the substrate as well as by thermodynamic, kinetic and growth parameters. This leads to a rich phenomenology of growth patterns and defects. E.g. the island shapes can become dendritic, compact hexagons, butterflies and more. Recently, it has been shown that the shapes can be simulated by an anisotropic phase field model (PF). Thus, carefully choosing anisotropic diffusion and step energies dependent on the substrate, the graphene island shapes seen on different Cu substrates could be reproduced.
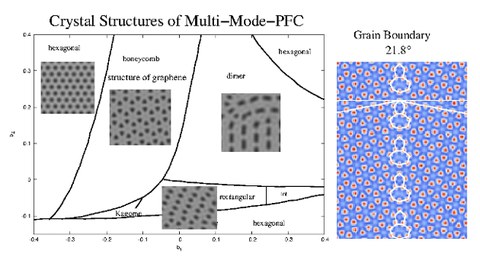
We propose to model graphene epitaxy by an extended phase field crystal model. In an PFC model, the anisotropy of the step energy and diffusion is implicitly controlled due to the interaction to the substrate. In order to model the graphene structure with PFC, the multi-mode extension for PFC has to be used (MMPFC). In MMPFC a lot of different two dimensional crystal structures can be modeled, e.g. hexagonal, honeycomb and rectangular structures. The description of the graphene as a density wave leads to a natural inclusion of defects in the graphene and elastic stress due to the coupling to the substrate.
Furthermore, MMPFC has to be extended to a model including the vapour phase (VMMPFC) in order to model and control the step of the grain in more details. Our task is to define the modeling parameters in a consistent way dependent on the interaction strength of graphene with the substrate.
Thus, we hope to derive a model description of the epitaxial growth of graphene, where all the anisotropy is described implicitly by the structure and coupling of the substrate to the graphene and has not to be chosen explicitly as in the PF model.
Project duration: 10/2015 - 09/2018
Funded by: ESF young researchers group "Computer Simulations for Materials Design" (CoSiMa) proposed by the Dresden Center for Computational Materials Science (DCMS), which is supported by the support-the-best program within the institutional concept of TU Dresden