Surface Vector and Tensor Fields
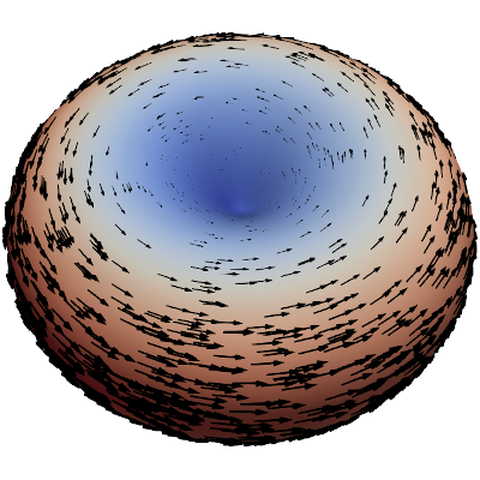
Circular flow field on biconcave shape
The discretization of vector- and tensor-valued PDEs on curved hypersurfaces poses methodological and analytical challenges compared to scalar-valued equations. One reason is the tight coupling between the tensor field components and geometric properties of the surface, such as its local curvature. The representation of the tensor fields is an issue that plays a role in the numerical implementation. Either we consider the surface as embedded in a surrounding space and represent the tensor fields as part of this embedding, or we look for a more intrinsic description of the surface and the fields.
While extrinsic models often allow a simple construction of a conventional numerical method, these may include additional stabilization terms due to their degeneracy. The resulting descriptions are often nonconformal, leading to more involved numerical analysis. Intrinsic methods, on the other hand, often have a very nice theoretical basis, but are often more difficult to implement in code.
Research Focus
The research project focuses on the development of numerical schemes for vector and tensor fields on surfaces and the comparison of these methods. Targeting stationary vs. moving surfaces raises the issue of which method to use in which situation. All approaches have some limitations and some areas where they are advantageous.
These abstract methods are then applied to applications involving vector fields, such as surface fluid flow or polarization fields for surface activity, and tensor fields, such as liquid crystal monomers attached to fluid interfaces or surface stresses in fluid mechanics.
Student projects and thesis topics
- Reconstruction of higher-order normal fields on discretized surfaces.
- Analysis of the discrete surfaces of even polynomial order.
- Discretization of the surface Stokes equation with surface finite elements and its numerical analysis.
- Development of discretization methods for subspaces of surface tensor fields, like symmetric tensors, trace-less tensors, Q-tensors, or nQ-tensors.
- Intrinsic surface finite element methods for tangential vector fields.
Publications
- H. Hardering and S. Praetorius. Tangential errors of tensor surface finite elements. IMA Journal of Numerical Analysis, 43(3), May 2023, pages 1543–1585, [doi] [arXiv]
- P. Brandner, T. Jankuhn, S. Praetorius, A. Reusken, and A. Voigt. Finite element discretization methods for velocity-pressure and stream function formulations of surface Stokes equations. SIAM Journal on Scientific Computing, 44(4), 2022, [doi] [arxiv]
- S. Praetorius and F. Stenger. Dune-curvedgrid - a dune module for surface parametrization. Archive of Numerical Software, 6(1), 2022, [doi] [arxiv]
- S. Praetorius, A. Voigt, R. Witkowski, and H. Löwen. Active crystals on a sphere. Phys. Rev. E, 97:052615, May 2018. [doi] [arxiv]
- I. Nitschke, M. Nestler, S. Praetorius, H. Löwen, and A. Voigt. Nematic liquid crystals on curved surfaces - a thin film limit. Proc. Math. Phys. Eng. Sci. 474(2214):20170686, June 2018. [doi] [arxiv]
- M. Nestler, I. Nitschke, S. Praetorius, and A. Voigt. Orientational order on surfaces - the coupling of topology, geometry, and dynamics. J. Nonlinear Sci., July 2017. [doi] [arxiv]
- K. Padberg-Gehle, S. Reuther, S. Praetorius, and A. Voigt. Transfer Operator-Based Extraction of Coherent Features on Surfaces, pages 283-297. Springer International Publishing, June 2017. [doi]
