(Amplitude) Phase-Field Crystal model
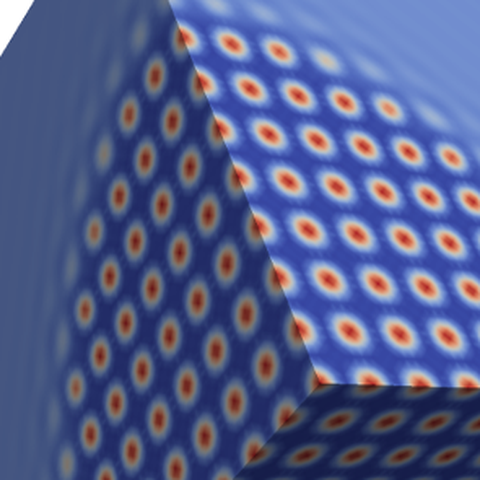
Coexistence of two phases - crystalline and liquid - within the phase-field crystal model in a three dimensional setup.
The Phase-Field Crystal (PFC) model describes the evolution of a particle density of spherical colloidal particles in the overdamped limit, based on an expansion of the interaction part of a Hemlholtz free energy. The lowest order PFC equation is given by a 6th order nonlinear PDE.
In an early work, we studied linear solvers and preconditioners for the linear systems arising from a FEM discretization of the PFC equation as a coupled system of second-order equations. The resulting block preconditioner solves the equations much more efficiently than many previously known approaches. It allows the handling of large 3D structures in a sequential or parallel computing environment.
Research focus
The PFC model not only has interesting applications, e.g. in materials science as a time-diffusive replacement for molecular dynamics methods, but it is also a challenging numerical problem as a Wasserstein or H-1 gradient flow of the Swift-Hohenberg energy functional. We also consider the equation in combination with a coarse-grained approach, the Amplitude Phase-Field Crystal model. The development of efficient numerical methods for both approaches and even a numerical scheme combining both equations of different time and length scales is the focus of this project.
Student projects
- Discretization of the Amplitude Phase-Field Crystal Equation [details]
Publications
-
S. Praetorius, M. Salvalaglio, and A. Voigt. An efficient numerical framework for the amplitude expansion of the phase-field crystal model. Modelling and Simulation in Materials Science and Engineering, 27(4):044004, 2019. [doi] [arxiv]
- S. Praetorius and A. Voigt, Development and Analysis of a Block-Preconditioner for the Phase-Field Crystal Equation, In SIAM J. Sci. Comp., Vol. 37 (3), pp. B425–B451, 2015. [doi] [bibtex]
