Discrete element modeling of concrete structures
Table of contents
Project data
|
Titel | Title |
Report in the annual report 2019
2D SIMULATION OF CONCRETE FRACTURE WITH DEM
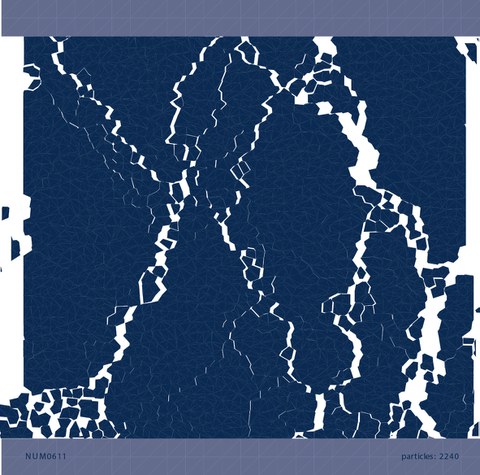
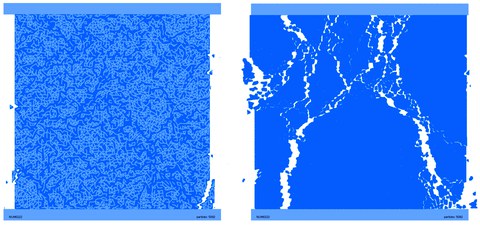
Crack pattern in the simulation of a virtual concrete specimen of 2,240 particles
Using numerical simulation based on the Discrete Element Method (DEM), concrete fracture and concrete failure phenomena are investigated in this project. While single elements resulting from the discretisation of the concrete specimen are only a calculation tool in continuum-based simulations, the single elements represent actually separate concrete particles being able to move kinematically independently in discrete methods or particles-based methods such as DEM. Furthermore, in continuum-based methods, steady fields are an implicit presupposition and, therefore, no gaps and no gaping joints may exist. In contrast, in the DEM, adjacent particles can also move apart from each other and do not need to be permanently connected to their neighbours. This basic approach allows the simulation of actual cracks. A crack that arises during the simulation is discrete, and a crack means an absence of concrete material, i. e. a gap or defect in the concrete. This property of the DEM, that cracks are discrete per se and can be simulated directly, is an essential reason for the choice of this simulation method. A further reason is the inherently contained complexity. Using a great number of particles, that differ only slightly due to random particle generation, but that are principally equal to each other, the complexity and the statistic character arise just due to the interaction of the particles. It means, that two simulations with exactly equal simulation parameters and only with two slightly varying particle ensembles lead to similar, but slightly different results. This mirrors the observations of real laboratory experiments. The same here – no two concrete specimens can have the exact same positions of aggregate, and the fracture patterns of two specimens show statistical deviation at the end of the experiment.
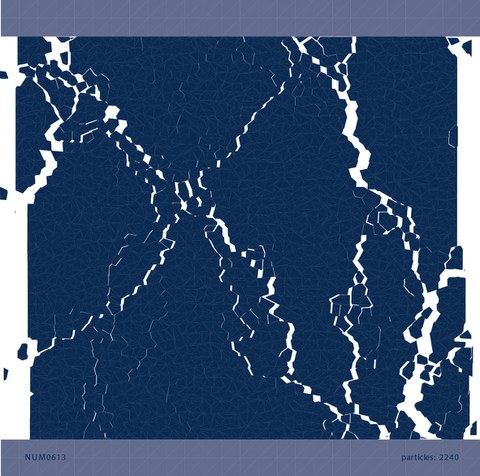
Crack pattern in the simulation of a virtual concrete specimen of 2,240 particles and with slightly varying particle positions
To get the statistical character out of the interaction of “great” many particles, the number of particles does not need not to be that big. Even a number of 2,200 particles is enough to achieve statistically varying results of two different ensembles, i. e. of two test specimen representations of the same concrete charge. The crack patterns of two ensembles with 2,240 particles, that differ only in the particle position due to random particle generation, but have exactly the same simulation parameters beside that, are similar, but slightly differ in the particular crack position – just like in real laboratory experiments, too.
Report in the annual report 2018
DISCRET ELEMENT SIMULATION OF CONCRETE FAILURE
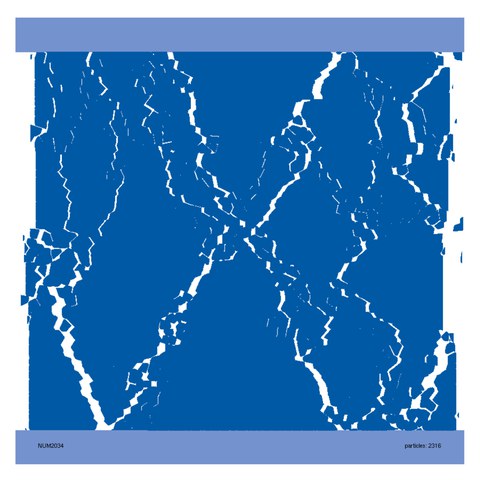
Rissmuster eines Betonprobenkörpers
Concrete fracture phenomena are investigated in this project, specially focusing on the investigation of their statistically varying character. In order to study the matter of failure mechanisms, crack propagation and damage evolution, a two-dimensional numerical simulation based on the Discrete Element Method (DEM) is used for the analysis of concrete behaviour under compression load. Crack patterns, crack initiation and damage evolution are analysed. The cracks are discrete just as in real laboratory experiments. The cracks arise due to the interaction of the concrete particle elements and without the predefinition of any crack zones or crack elements.
This simulation employs a combination of essential elements such as a contact approach including an overlap area, detection of new particle contacts during the simulation instead of neighbouring lists and particles with arbitrarily polygonal shape and, furthermore, the inclusion of statistically varying properties. While the comparison of simulation results to the experimental results was addressed in detail in previous works, the focus of this year’s research is laid on the presentation and description of theoretical background including statistical aspects. Two statistical approaches are used in the simulation. First, randomly varying particle geometries and particle positions are generated due to a random factor used during particle generation. Second, a Weibull-distribution for maximum elongation of the (optional) cohesion linkages is used. The statistic approach is rather elementary instead of elaborate; and there are no macroscopic mechanical effects added to the model. Based on this, there are the complexity of the particle ensemble and the interaction of many simple particles letting macroscopic effects such as cracks and crack patterns emerge.
Using statistical variations of geometrical and material properties, it is shown that even an elementary statistical approach leads to the evolution of statistically varying crack patterns. It shows that the DEM is a suitable approach to study failure and fracture processes.
Report in the year book 2017
DISCRETE ELEMENT SIMULATION OF CONCRETE FAILURE

Crack patterns of concrete specimens under higher loading velocities
The failure behaviour of concrete is investigated using a numerical simulation based on discrete element method (DEM) in this project. The aim is particularly to analyse the failure and fracture including the crack evolution rather than the load bearing behaviour within safe static loading ranges. For this purpose, a discrete simulation is especially suitable since cracks are an inherent part of the simulation method. In the simulation, the concrete specimen is represented by an ensemble of single particles. A discrete approach fundamentally differs from continuous approaches to that effect that equations of motion of the single particles are kinematically independent and that discontinuities are an inherent property of the method.
In the numerical simulation, the concrete specimens are generated in that way that they differ regarding statistically varying positions of the single particles. This corresponds to different specimens of the same manufacturing charge in real laboratory experiments, since particular positions of aggregate pieces and matrix underlie statistic variations in laboratory experiments, too.
In the simulation, different particle generations with the same further parameters are calculated, similarly to different specimens of the same charge in real experiments. Furthermore, exactly the same particle ensembles are calculated until ultimate load and failure several times – under lower and under higher loading velocities. The opportunity to analyse one and the same specimen under different loading conditions is an advantage of the simulation versus the laboratory experiment. Two relevant results can be seen in the figures. Firstly, it is shown that the crack patterns of different repre-sentations of the same charge are principally similar but differ in the details of their particular crack positions. This is the same as in the laboratory experiments, where the cracks of two specimens are never exactly identical. Secondly, the figures show that the similarity of the crack patterns increases with increasing loading velocities and also that branching of the cracks is higher.
Report in the year book 2016
Simulation of concrete components using discrete element method
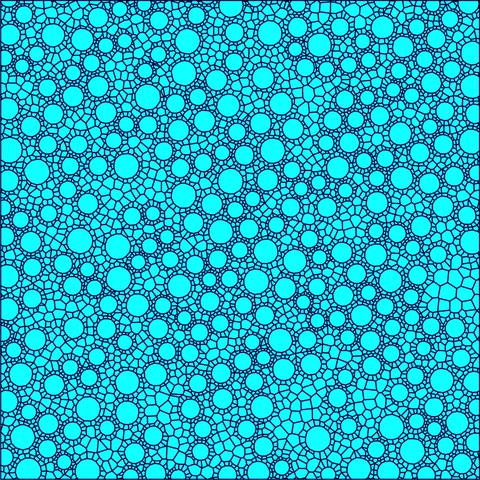
Substructure for aggregate and hydrated cement – ensemble of polygonal particles of different sizes
Within a numerical simulation using the discrete element method (DEM), the concrete specimen is represented by an ensemble of single particles. Using a discrete approach for the numerical model, the equations of motion of the single particles are kinematically independent, and discontinuities are an inherent property of the simulation method. Especially due to the last-mentioned property, discrete approaches are favorable for the simulation of cracks and the investigation of failure processes. With regard to the calculation of the interaction forces of contacting particles, a convex shape of the particles is necessary.
In order to consider both convex and concave specimen geometries, particles on a concave corner are divided into subparticles during particle generation. This is to preserve the convex shape of the particles themselves even in the case of concave geometries of the whole test specimen. Polygonal particles are used in this project. Due to this, the simulation is limited to only two dimensions up to now, although the simulation is principally designed with three dimensions, of course. Another simulation has been developed at our institute, it is a simulation using spherical particles. For this simulation, all three dimensions can be used.
In 2016, the particle generation and the generation of a substructure was one of the working points. The aim is to represent the different concrete components such as aggregate and hydrated cement. Therefore, the two simulations mentioned above are combined. At first, a mixture of grains of different diameters is simulated using the simulation with spherical particles. Then, a modified Voronoi-tesselation is done with the midpoints of the particles representing the aggregate. For the modification, helping points are places around the aggregate particles in randomly varying distances in order to get much smaller and also randomly or statistically varying particles which represent the hydrated cement.
This leads to particles of different sizes, that are polygonally bounded though they originate from spherical particles. The polygonal shape is required to get a dense particle ensemble. Furthermore the convexity is required for the force calculation.
Report in the year book 2014
DEM 2D – Fracture phenomena in concrete
Intact cohesion bars (light blue) and broken cohesion bars (dark blue) show in advance (left) the later evolution of macro-cracks during post-peak behaviour (right)
To guarantee the safety and load bearing capacity of constructions in building practice, a sufficient margin always needs to be considered. In contrast, the point of failure and fracture in experiments or numerical simulations is specifically targeted in basic research. When do micro-cracks arise? How do they increase up to macro-cracks? How homogeneous or heterogeneous does the damage evolution proceed? To what amount can the load still be increased from the point of damage initiation to that of entire failure? What kind of crack pattern or failure pattern shows a concrete specimen at total collapse? Which damage mechanisms can be identified? Can the effects of the damage evolution be already seen at its beginning or initiation? – In order to answer such or similar questions the concrete failure behaviour is investigated using a two-dimensional numerical simulation based on the discrete element method (DEM). Another aim of this approach is to generalize the applicability of such simulations.
In fact, the concrete specimen is represented by an ensemble of discrete particles. The particles can dynamically move independently from each other. When two particles collide and penetrate each other in an assumed overlap area, a repulsive force is calculated based on this overlap area. In doing so and taking into account the interaction of all particles of the ensemble, compressive forces are transmitted from the loading plate to the support plate. Such a numerical model is useful for the description of brittle materials under mostly compressive load, as is the case for concrete. In order to describe tensile cohesion forces beside the mentioned compressive contact forces, an additional lattice of springs or bars is modelled. These bars can optionally transmit normal forces or normal forces and bending loads.
In the simulation, the principal force flow within the concrete specimen can be depicted by means of bars of different thickness. The thickness of the bars represents the amount of force of the respective bar. Especially at the beginning of the loading procedure, it can be shown that the force flow, originating from the loading plate, has not completely reached the supporting plate at an early loading point. Furthermore, the broken cohesion bars show the later crack and failure pattern which was already observed at an earlier point in time during the loading increase.
