Description
Keywords:
unconfined groundwater flow, free boundary problem, analytic solution, gorundwater-surface water interaction
Objectives
The analytic solution of the 2d Laplace equation for steady unconfined groundwater flow in a deep homogeneous and isotropic aquifer derived by Schmitz and Edenhofer [1, 2] is to be extended and applied to hydological scenarios. The main objectives are:
- to invoke inner sources and sinks (Poissons euqation)
- to consider shallow aquifers
- to incorporate vertical boundary conditions
- to derive formulas for the solution of the inverse problem, to determine the recharge rate as a consequence of a given groundwater table
- the semi-analytic solution of the transient problem
- to consider geometrically arbitrary lower boundaries
The derived solution is to be implemented in the software package TEXAS2D and in a software module, that can be linked with other groundwater modeling tools.
Beside the extension of the mathematical solution, its hydrological and engineering interpretation and application examples include:
- application of the inverse computation for determining the exchange rate betweenn groundwater and surface water
- Sensitivity analysis, comparison with common groundwater models, experimental and field data
- incoorporation of the mathematical solution into an user friendly software tool
Methods
A homogeneous isotropic aquifer with a phreatic surface and an impermeable horizontal stratum beneath is influenced by superficial recharge / drainage, described by a function N(x) and inner sources / sinks. We consider a vertical cut of the aquifer.
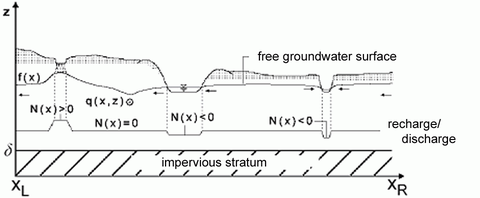
Info
The basic idea of the analytic solution of the corresponding free boundary problem is the conformal transformation of the aquifer to a parallel strip. The transformed boundary problem is solved on the parallel strip. Thus we achieve information about the phreatic groundwater surface and can determine the inverse transformation back to the real aquifer.

A solution of the boundary value problem
The essential key to both the solution of the steady and the inverse groundwater problem, is to derive a relation between the recharge function N and the phreatic surface f on the parallel strip. The same relation allows for a semi-analytic solution of the transient problem. Thus, the time derivative of the surface is computed analytically, while the groundwater surface is updated by means of a numerical procedure.

Solution of the unsteady processes
Lateral boundaries of the domain are expressed by appropriate arrangements of inner sources / sinks. Details about the mathematical algorithm and its implementation in the software tool TEXAS2D can be found in the TEXAS2D Manual. The PhD-Thesis of J. Weber, Centre of Mathematics, TU Munich 2003 contains an extensive theoretical foundation.
The inverse computation, i.e. determining the recharge rate as a consequence of a given groundwater surface completely opens new possibilities for detecting exchange rates.
Results
The solutions of the direct steady and, to some extent also, the inverse groundwater problem are already implemented in the software tool TEXAS 2D (Tool for EXact Analytic Solution of 2D-groundwater flow problems). The complete inverse solution and the semi-analytic algorithm for transient problems in shallow aquifers are currently being tested and analysed.
