Multi-scale failure analysis with polymorphic uncertainties for optimal design of rotor blades
A continuation of the topic is currently taking place in the second project phase.
Main goals are the identification of polymorphic uncertainties in the structural design of rotor blades and to develop multi-scale (in space and time) non-deterministic models and efficient numerical approaches, which are able to incorporate these uncertainties into a typical „design – testing – implementation – maintenance“ chain.
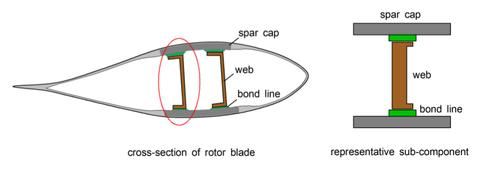
Cross-sections of a rotor blade and a representative sub-component for investigation of adhesive joints
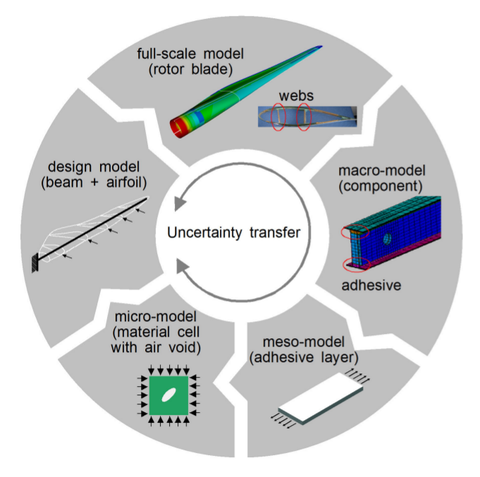
Multi-scale analysis for rotor blades
- Identification and description of polymorphic uncertainties in the design of rotor blades. (Fuzzy and probabilistic methods)
- Description of full-scale model and decomposition into structural components with polymorphic uncertainties and development of FE simulations. (FE, Monte Carlo, stochastic FE)
- Uncertainty modelling in macroscopic component model, in particular glue layer with homogenized material and imprecise random strength and stiffness. (multiscale FE, numerical upscaling, model reduction, tensor methods)
- Multi-scale fatigue modelling.
- Modelling and simulation of non-deterministic mesoscopic glue layer in adhesive contact.
- Development of complete component simulator.
- Validation and integration of component and full models.
Summary of the first funding phase
The quality of adhesive bonds in rotor blades of wind turbines significantly affects the overall structural integrity and reliability. Inaccuracies and imperfections due to manufacturing processes and environmental conditions can lead to insufficient bonding of the structural components and also to critical air voids in the adhesive bonds. Experimental and numerical investigations have been conducted in the first funding period for the latter one.
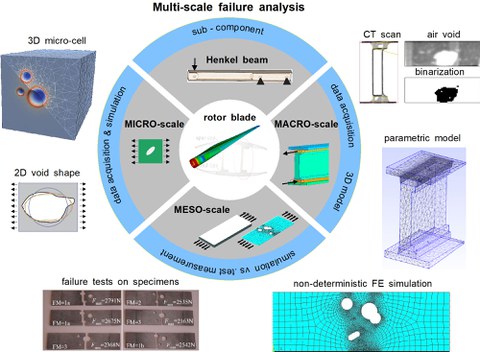
Multi-scale failure analysis concept under polymorphic uncertainties
A multiscale model has been defined consisting of a macro-, meso- and micro-scale. The Henkel beam has been developed by Fraunhofer IWES as a representative subcomponent for investigations of adhesive bonds in rotor blades and serves as macro model. Holed 2D and 3D domains have been used on meso-scale as surrogate models for adhesive bonds and single cells with air void within the adhesive bond define the micro-scale.
Air inclusions could be identified by computertomographic scans of several Henkel beams. The non-destructive testing (NDT) data have been analyzed, processed and used for modeling uncertain parameters like amount, location, shape and size. A general concept regarding data acquisition, modelling, processing and assimilation have been developed in the framework of complex A. Additionally, it has been applied in cooperation with other research groups within the SPP 1886 on multiple engineering problems.
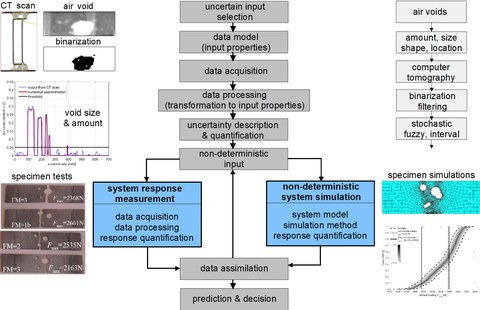
General approach to data acquisition, modelling and assimilation in engineering problems
The acquired data have been integrated in the parametric 3D Henkel beam model leading to FE simulations under polymorphic uncertainties. Probabilistic as well as non-probabilistic methods have been used. The simultaneous consideration of stochastic, interval and fuzzy variables has required the development and application of efficient numerical techniques. The adhesive bonds with air voids as mesomodel have been extracted by Guyan reduction from the entire model. Parametric domain decomposition methods based on Schwarz alternating method, Schur complement method or FETI-DP method have been implemented successfully. As a consequence, the air voids with polymorphic uncertain parameters have been decoupled on micro scale resulting in a very efiicient multiscale Henkel beam model. Nevertheless, it has been suitable to study different approximation methods with regard to polymorphic uncertainties. Artificial neural networks (ANNs) have been defined with high accuracy compared to a computational costly reference solution for the described problem.
In order to validate the examined numerical methods and models described above, a representative meso-scale problem has been investigated experimentally. For this purpose, a series of Plexiglas strips with similar properties have been fabricated and then tested under uniaxial tensile forces. Despite of an identical setup, different failure mechanisms with associated ultimate loads could be identified and are subject of inherent uncertainties which have been included in numerical simulations. The experimental results have been captured qualitatively and quantitatively by the numerical models. Furthermore, ANNs have been used to reduce the computational costs of finite element simulations and have produced similar results with negligible deviations. The uncertain parameters have been defined as stochastic and/or fuzzy variables. It has been shown that the same ANNs could be applied satisfactorily independent of the type of uncertain data.
Essential project findings
-
Identification of air void inclusions in adhesive bonds by non-destructive testing (NDT)
-
Development of a general concept regarding data acquisition, modelling, processing and assimilation in engineering problems
-
Definition of a multiscale-model consisting of macro-, meso- and micro-scale
-
Development of a parametric 3D Henkel beam model and finite element simulations under polymorphic uncertainties
-
Developement of efficient numerical tools for high-dimensional problems, including adaptive parameteric domain decomposition or hierachical low-rank tensor formats
-
Realization of artificial neural networks (ANNs) and application to problems with uncertain data, especially to quantify and locate stress concentrations in holed domains
-
Comparison of numerical and experimental investigations on a representative surrogate problem on meso-scale
-
Investigations of concepts for assessment, decision making and decision improvement under polymorphic uncertainties

Robert Gruhlke
Robert Gruhlke
Weierstraß-Institut

M. Sc. Martin Drieschner
M.Sc. Martin Drieschner
Technische Universität Berlin
Fachgebiet Statik und Dynamik
- Publication 1



