Modelling of vibro-acoustic systems with polymorphic uncertainties and its application to tyre road noise
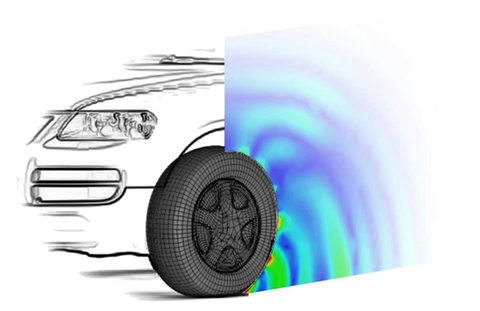
Sound radiation of a tyre
The acoustic quality of products gains an increasing importance in everyday life. Unfortunately, rather similar looking products of the same manufacturing process tend to show a high variation of their acoustic properties, which is based on geometry deviations or varying material parameters. Therefore, a confidence interval instead of one deterministic solution needs to be determined in order to allow for an early optimisation in the development of new vibro-acoustic systems. Doing so, it should be noticed that different characteristics of uncertainty may occur, namely variability, imprecision, and incompleteness. Consequently, an incorporation of just one characteristic is unrewarding. Instead, the polymorphic uncertainties of the quantities need to be handled, which is the general objective of this proposal. A representative technical example, strongly depending on the behaviour mentioned before, is tyre road noise. Therefore, this problem has been chosen to demonstrate the design process taking into account polymorphic uncertainties. Up to now, only deterministic numerical computations have been developed to predict tyre road noise, by other researchers as well as by the applicant. In a close collaboration between the latter one and the Chalmers University, Gothenburg, particularly in the project Leiser Straßenverkehr 3 (supported by BMWi), a very efficient and precise model for analysing the structural behaviour of tyres and their related sound emission could be developed. Within the current proposal this combined – but so far deterministic - numerical model shall be extended in order to introduce the polymorphic uncertainties to tyre road noise simulations. The treatment of multiphysical results, which rely on polymorphic uncertainties, has to be incorporated in the new model. The planned optimisation with respect to significant output parameters, such as the radiated noise and the rolling resistance, requires highly efficient numerical methods as well as a reduction of the problem dimension. Hence, the Fast Multipole Boundary Element Method is used for the sound radiation, while a Waveguide Finite Element Method is employed to represent the structural model. Although these two methods are very efficient already, a further reduction of the computational effort shall be established by a new metamodel, which promises an efficient way to handle the rather complex multi-objective optimisation also planned in the current proposal.

Prof. von Estorff
Prof. Dr.-Ing. Otto von Estorff
TU Hamburg-Harburg
Institut für Modellierung und Berechnung

M. Sc. Thomas Kohlsche
M. Sc. Thomas Kohlsche
TU Hamburg-Harburg
Institut für Modellierung und Berechnung
